Laser Link in Space

Data Transfer

Two satellites in low Earth orbit send and receive data at 20 Mbps using infrared lasers. To do this, the satellites use a scheme known as pulse-position-modulation, which encodes information into the arrival time of a burst of light. If you are unfamiliar with this scheme, imagine the song Stayin’ Alive by the Bee Gees. You can count along to this song’s four-beat rhythm as 1-2-3-4, 1-2-3-4, and so on. If you and a friend are listening to the song together, you can use the rhythm to send each other messages. You start by agreeing on the meaning of each beat ahead of time: if you wink on the first beat of a measure, that means 1, if you wink on the second, that means 2, winking on the third means 3, and you can use complementary logic from here. Now, you and your friend can sit across from each other listening to Stayin’ Alive and winking to send strings of numbers. This allows you to transfer files by transferring them two bits at a time, mapping 00, 01, 10, and 11 to each of the four beats in a measure. Stayin’ Alive is nominally at 104 beats-per-minute. At two bits per beat, it would take us about two weeks to transfer the complete 512 kB file size of Pokémon Red Version*. If we double the speed at which Stayin’ Alive is playing, we double the maximum rate at which we can send data. This is why having a rapidly oscillating CSAC is so useful for sending data. The ticking of the CSAC oscillator acts just like the rhythm of your song. You may anticipate an issue with progressively faster oscillators or faster renditions of the Bee Gees. As the beats get closer together, it becomes easier to confuse adjacent numbers, and small deviations in the rhythm can cause errors in the transmission. This is why the CSAC’s frequency stability is crucial to sending information.

Aboard the CLICK mission, we use two chip-scale atomic clocks to provide rhythm to the satellites. Instead of winking at each other, the satellites send a pulse of laser light. The satellites keep time relative to each other like the rhythm of a song. Depending on the beat at which a satellite receives a pulse of light, the satellite records different information.

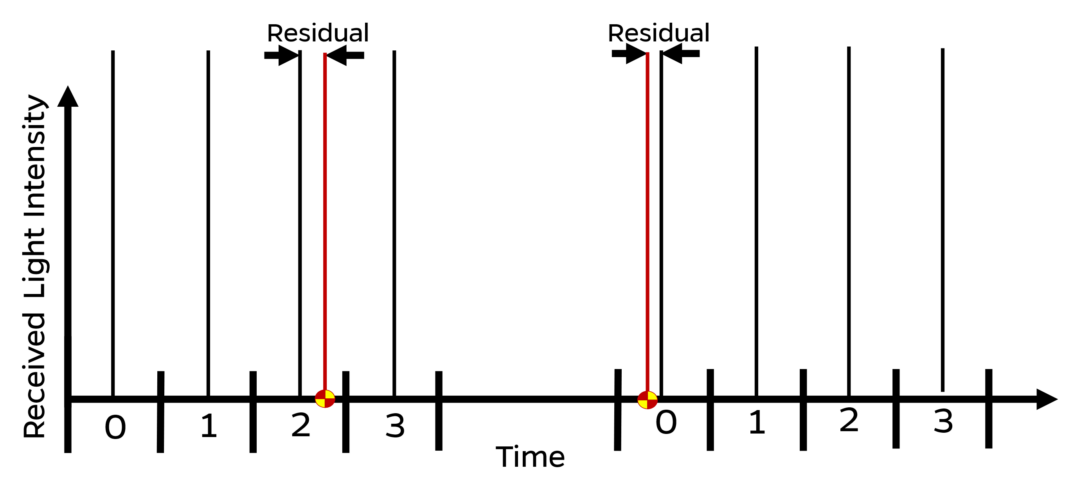
Figure 1. Pulse Position Modulation Diagram.

The vertical black bars represent the center of the time slot which encodes the value written on the underside of the ‘Time’ axis. The thick bars on either side of the encoded value labels indicate the edges of the time slots. You may notice there is a large gap between the two sets of symbol values. This ‘guard time’ helps reduce the chances of confusing pulses. In the song analogy, this would be akin to saying two rest beats must follow each four-beat measure before starting the new measure: 1-2-3-4-rest-rest-1-2-3-4-rest-rest-1-2-3-4.

Clock Correction & Time Transfer

Now that you have a tangible example of what is happening in pulse position modulation, let us consider a scenario which illustrates the difficulty faced when sending information between devices with two different clocks rather than the same clock. You and your friend load Stayin’ Alive onto two old iPods**, put in earbuds, and try to hit play at the same time. Due to wear on the devices, the iPods randomly lag and skip in the song. As you and your friend begin winking numbers to each other, you notice when she falls behind your rhythm and when she gets ahead of your rhythm. If you keep a running tally of how far your friend’s rhythm falls behind or gets ahead of your rhythm, you can estimate where she is in the song at any point in time by taking your position in the song and adding on the accumulated difference between his point in the song and your point in the song. This is known as clock correction. On board the two CLICK satellites, the clocks drift relative to one another like the songs on the lagging and skipping iPods do. We know when pulses are supposed to arrive to encode a number, but we have a window around that arrival time wherein we can measure how behind or ahead the transmitter’s clock is. If the pulse arrives slightly early, we record that the transmitter’s clock is ahead of ours. If the pulse arrives slightly late, we record that the transmitter’s clock is behind ours. If we have a good estimate of the offset between the transmitter and receiver clocks, the transmitter can record its current time and send it to the receiver via the laser link. The receiver can read that time, note the time on its internal clock, account for the known offset between the receiver and the transmitter, and compute how long the message took to arrive. If we know the speed of light, we can use the message’s time-of-flight to measure the distance between the satellites. The precision of our distance estimate is equal to the precision of our time-of-flight estimate multiplied by the speed of light. CLICK’s 50 centimeter ranging precision thus requires 1.66 nanosecond timing precision. We can measure the clock offset at an instant in time using the Residual value shown in Figure 1. We lowpass filter the residual measurements to get rid of transient white noise in the reading and recover the underlying drift between the clocks.

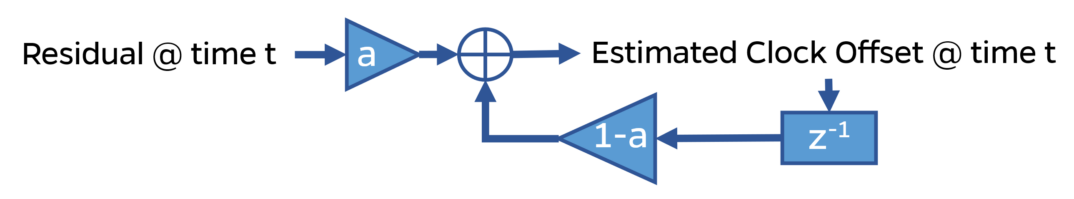
Figure 2. Low pass filtering the clock offsets.

CLICK uses SA45S chip-scale atomic clocks aboard each satellite enable this precise distance measurement and high data rate communications. The CSACs’ highly stable 10 MHz oscillation rate allows the satellites to measure the time-of-flight of laser light to fine precision and allows the satellites to discriminate between changes in pulse arrival time on the scale nanoseconds.

*This claim comes from thegamer.com, a source whose reputability I have not verified and whose claims regarding Pokémon file sizes are contradicted elsewhere. The point is that a slow oscillator takes an eternity to transfer even a small amount of data.

**This was a type of smart phone (circa 2001) which only played music.